![]()
Therefore, it’s essential to ensure your students approach them with confidence. This post will help you do exactly that, offering some practical suggestions as to how some objectives could be taught in the classroom.
The word fraction has very little meaning to students. The word fraction comes from the Latin ‘fractio’ which means ‘a break’ especially into pieces.
I would suggest that pupils are explicitly taught this as it helps to provide a mental model as to what we mean by ‘a fraction’. A break in a whole. This is especially important for children in Year 3 to grasp as much of the fraction work done in that year group is the breaking of wholes into parts.
Fractions are a core part of the primary maths curriculum, being introduced in Key Stage 1 with simple fractions of amounts and ordering fractions.
As they move into Key Stage 2 however, pupils will be asked to use fractions in ever more complex ways, from learning to divide and multiply fractions to completing fraction word problems.
As anyone who has gone through the National Curriculum for maths will know, the objectives related to fractions makes up a large proportion of the entire curriculum.
The teaching of fractions begins all the way back in Year 1 where students are expected to find fractions of shapes and appropriate quantities. In Year 2, they must be familiar with ⅓, ¼, ²⁄₄ , and ¾ of suitable objects, lengths, shapes and appropriate quantities and associate simple fractions with division, e.g. ½ of 6 = 3. Finally, they must be aware that ²⁄₄, and ½ are equivalent.
As I hope I have stressed in all the other blogs in this series, test the prerequisites and start from there. If students are unable to do the above, then it is unlikely they will be able to grasp fractions in KS2 maths.
Fractions is usually a topic that fills some teachers with dread. I believe this to be because of three reasons:
As professionals, we should be seeking out the opportunities available to us to improve our practice and break this cycle. You may wish to read our article on fraction games to make the process of teaching fractions more engaging and dynamic for your pupils.
A key step in pupils’ understanding of fractions is that they know early on that a fraction is not bound by a whole and has its own sense of ‘numberness’. Cuisenaire rods provide an excellent means of doing this.
You could ask students to find half the purple rod.
They will quickly identify that red is half of the purple rod and place it like this, underneath the purple.
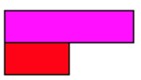
Ask them to find the other half of purple. Without difficulty students will be able to find another red rod and place it down.
The teacher could then place a white rod under the red and ask for the relationship between the white and the red. Probing questions such as ‘How many more white rods will I need to place down to be equivalent of a red rod?’ can be asked to move thinking forward.
They will come to the conclusion that 1 white is half of a red, which was half of the purple. This can be continued until a train of 4 white rods are placed under the train of red rods.
We have now built up a representation that demonstrated how 1 whole is equivalent to 2 halves, which is equivalent to 4 quarters and the idea that a quarter is half of a half. I would have this written out as 1 = ²⁄₂ = ⁴⁄₄. Students should then be asked to make some conjectures. Hopefully seeing that when the denominator and numerator are equal, the fractions are equivalent to one whole.
Next, we could ask students to place another half of purple down. Here the rods have a distinct advantage over commonly used visual representations, such as circles to represent pizzas.
If using pizza, and we ask students to get another half of a pizza, they could turn around, quite rightly, and claim that it cannot be done as there is no more pizza left. With rods, a child can easily understand that by adding another half, they would need to collect another red rod and place it at the end of the train of red rods, like so.
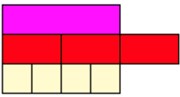
Now the students can understand that a fraction can denote a number >1. You may now wish to get students to conjecture how many more quarters are needed to be equivalent to three halves. They will see either through using more rods or identifying the pattern previously, that 2 more white rods (quarters) will be needed.
Teachers could now write that ³⁄₂r = ⁶⁄₄w. Finally, you could ask students to find just one other rod that is equivalent to ³⁄₂r = ⁶⁄₄w. After some experimenting, they will be able to place a light green rod under the train of white rods.

We can now express that 1lg = ³⁄₂r = ⁶⁄₄w.
Throughout the rest of this blog, I will demonstrate how Cuisenaire rods can be used to demonstrate other mathematical ideas with fractions
In the National Curriculum for maths in England, for each area of maths outlined, there is both a statutory requirement and a non-statutory requirement. The statutory requirements for fractions in KS2 maths are as follows:
Non-statutory notes and guidance:
For this lesson, I will look at the objective ‘Add and subtract fractions with the same denominator within one whole [for example, + = ]’ as this tends to be one that novice teachers can find quite tricky. As a prerequisite to this, it is important that students are familiar with the ideas expressed in the previous section.
Show an orange rod on the board. Tell students that is equivalent to one whole and we want to know how many white rods are equivalent to it.
Depending on your students’ exposure to Cuisenaire rods, they may be able to say that 10 whites are equivalent to orange immediately as, when we think of white as one, orange represents 10. Show this equivalence by placing the correct number of white rods in a train so that it is equivalent to the orange.
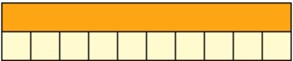
Students should be able to conjecture, if they are familiar with the ideas from the ‘theory’ sections that the white rods represent 10/10 which is equal to 1.
Once you are sure that all students in the class are comfortable with the idea, remove three white rods.

Students should be able to tell you that there were 10 white rods which we write as 10/10. Ask how many of those tenths have been taken away and the students will respond that that 3 have been taken away. It is appropriate here to remind students that three has not been taken away but rather 3/10ths – an important distinction.
As experts we can infer that another expert would say 3 in the full knowledge that what is being referred to is the tenths. We should come to no such conclusions when working with novices. We can then ask the final questions which is how many tenths are remaining. The answer being ⁷⁄₁₀.
We can write what has happened here in a formal method, perhaps repeating the process of taking the white rods away. ¹⁰⁄₁₀ – ³⁄₁₀ = ⁷⁄₁₀.
Next we may ask how we can go from ⁷⁄₁₀ to ⁹⁄₁₀. We can see from the model below that this would simply require the students to add an additional ²⁄₁₀.
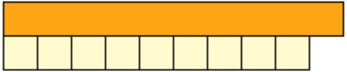
We can write what has happened here in a formal method, perhaps repeating the process of taking the white rods away. ⁷⁄₁₀ + ²⁄₁₀ = ⁹⁄₁₀.
This can be repeated over and over again with different factions within tenths and using different rods to represent 1 whole.

Fractions Intervention Pack
Download our intervention lessons to help your pupils develop their knowledge and understanding of fractions.
Word problems expected at this stage would be similar to the following:
Laura and Matt are eating a pizza. Laura eats ⁵⁄₁₂ of the pizza and Matt eats ⁴⁄₁₂ of the pizza. How much of the pizza was eaten?
This can be demonstrated using rods. The image below demonstrates ¹²⁄₁₂ which would represent the whole pizza.
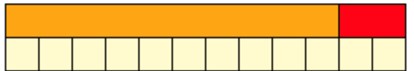
We can then remove the 5 white rods which would represent 5 slices of pizza Laura ate.
Then, we could remove a further 4 rods to show the number of pieces that were eaten by Matt. The remaining white rods demonstrate how much of the pizza is left.

An appropriate reasoning and problem-solving activity could be to look at what misconception a student may have on what has been studied.
Laura says ⁴⁄₇ + ²⁄₇ = ⁶⁄₇
Matt says ⁴⁄₇ + ²⁄₇ = ³⁄₇.
Who do you agree with and why?
A common misconception students fall into when adding or subtracting fraction for the first time is that both denominators must be added or subtracted.
When using the rods to introduce fractions, I have found that that misconception rarely occurs and when it does it is a useful diagnostic tool for a misconception about addition and subtraction.
That being that you can only add and subtract like items. Consider for a moment that you have 4 apples and 2 bananas. You cannot reasonably add these things together because they are very different. The only way to add these 2 quantities together is to frame them as ‘fruit’ and so you end up saying you have 6 pieces of fruit.
If students have this understanding, they quickly realise that adding unlike denominators is not possible. Therefore, Laura is correct.
Non-statutory notes and guidance:
For this part I want to focus on the following objective:
When looking at equivalent fractions, using fraction walls should be your first pedagogical choice. Every child should have one and, in an ideal world, know not to touch and fiddle with the pieces until you have told them to.
If you know students are not capable of this, it is best to let them have a play with them first and see what they produce. This always provides some interesting discussion points if you are observing what the students do and notice.
By this time, students should be familiar with simple equivalents like halves and quarters and with denominators up to 4, 10 only and 100 only. In this lesson we will extend their understanding of denominators up to and including 12.
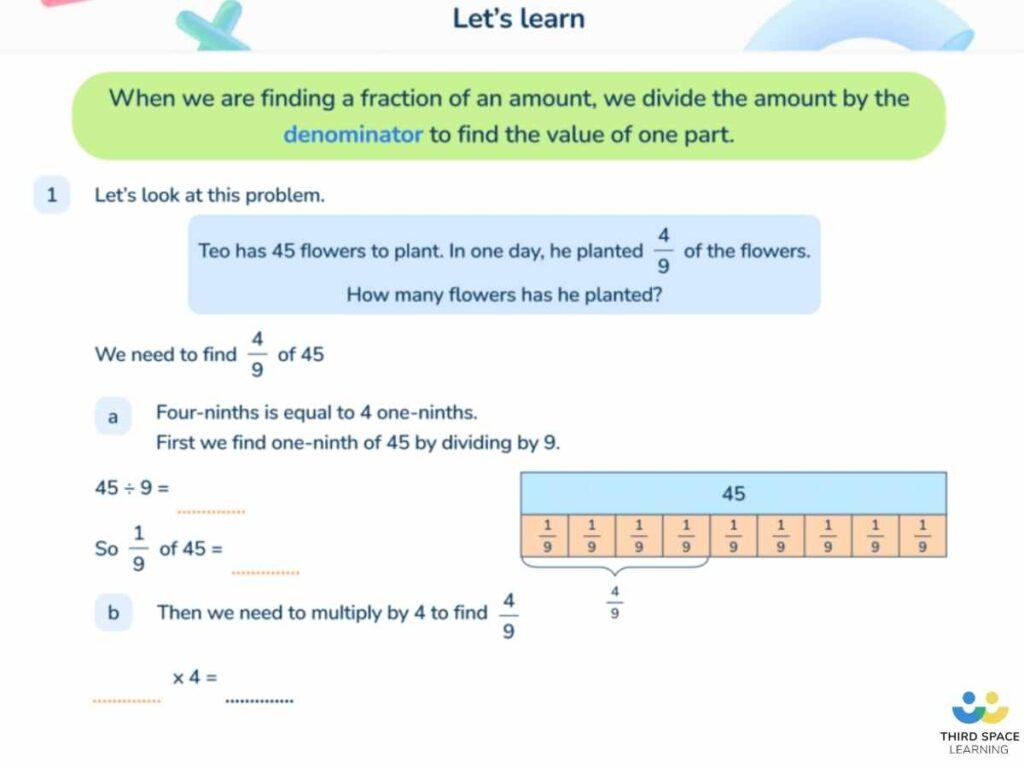
Explain what is meant by equivalent fractions. Here, students can use their knowledge of the words to see equivalent begins with a word similar to equal which means ‘the same’ and fractions they know means ‘a break’. So equivalent fractions are breaks which are the same.
Begin by asking the students to get ½ from their fraction wall.

Ask students place down the thirds and ask can you place the thirds underneath the ½ so that the total length of both bars are the same length. They will soon realise that they are not able to do this. Write ⅓ on the board.
Move onto quarters and ask them to do the same as with the thirds. It is important that pupils can see how the two blue blocks representing ¼ each is the same as ²⁄₄. They will tell you that it can be done and that ²⁄₄ are the same as ½.
Write down ²⁄₄ on the board in a separate area from ⅓. Ask if they can do the same with fifths. Again, they will find out that you cannot find an equivalent fraction of ½ with fifths. Write ⅕ on the board next to ⅓.
Ask them if it can be done with sixths. Very quickly the students will realise that it can be and they will have the following:
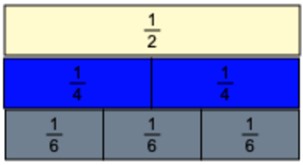
Write ³⁄₆ on the board where you have written ½ and ²⁄₄.
Repeat the above until you have gone through all the denominators through to 12. They will have the following:

And on your board you should have:
½, ²⁄₄, ³⁄₆, ⁴⁄₈, ⁵⁄₁₀ and ⁶⁄₁₂ together and ⅓, ⅕, ⅐, ⅑ and ¹⁄₁₁ together.
From here, you need to be directing and probing students’ thinking so that they can make some conjectures. Can they see that within the equivalent fractions for ½ that the numerator and denominator are both even numbers? Do they see that the numerator is half the denominator?
What conjectures can they make from this? Would ³⁸⁄₇₆ therefore be equivalent to half? And for the numbers on the other side, what can they deduce from that? Ah, when the denominators are odd then an equivalent fraction to ½ cannot be made through a fractional representation.
You could then guide and ask students to find other fractional equivalent for other fractions.
It is important to remember that we want the students thinking; not just placing different parts of the fraction wall down in a ‘have a go’ way. So asking students to conjecture each time they have found the fractional equivalents is so important. For 1/4 we would expect students to notice that only when a denominator is in the 4 times table will it contain an equivalent fraction.
The focus and outcome of this lesson should and always be for students to begin making these types of conjecture not to play around and build up the fraction wall. When teaching, you need to ensure that that is at the forefront of your mind. Carefully planned fraction questions are key here.
A typical word problem could be the following:
I know that ²⁄₄ is the same as ²⁄₂ because the numerators are the same. Is this statement correct or incorrect? Explain why.
Students could draw bars in their book to demonstrate this:

Or explain that when the numerators are the same, the larger the denominator the smaller the fraction.
A reasoning activity could be the following:
Using the numerals 1,2,4,5,8 and 10 find all the equivalent fractions.
This is where all that conjecturing done previously can come into play. The more of that the students have done, the more mathematical their approach to solving this would be.
They will not need to use trial and error with the support of a fraction wall, rather they can use what they know about the underlying relationships of fractions and equivalence. That said, ensuring that fraction walls are available to support learners is important.
You would expect students to find the following:
A popular approach to teaching fractions in this year is to use a fraction wall, pictured below:
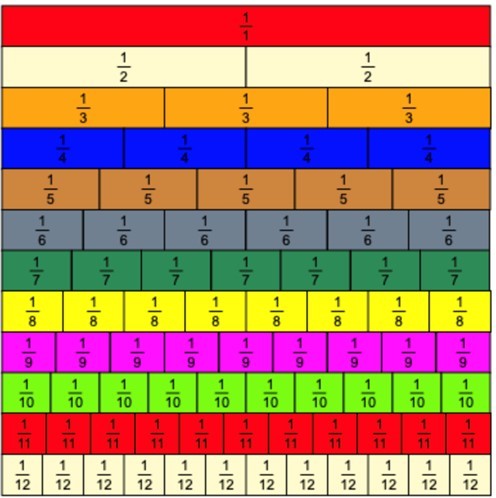
The fraction wall works in a slightly different way to Cuisenaire rods as I am sure you can see. While the rods help us see the numberness found within fractions, the fraction wall helps us to see that we can break one whole into many equal parts without being hindered by the physical size of the rod.
For example, it is impossible to demonstrate that ³⁄₃ is equivalent to ¹⁄₁ using Cuisenaire rods as the length of the rods would not match the value of the whole.
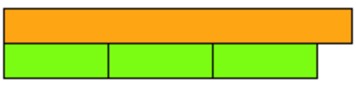
If I swapped out the value of the whole from the orange to the blue, we would then see that there are no 2 rods that could make the length of the blue.
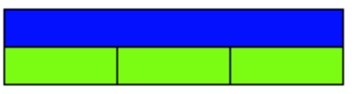
Here we can see that, in an attempt to represent ½, that the yellow rods are physically too big while the purple rods are physically too small.
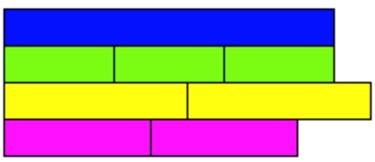
We are therefore, left with no choice other than to select the fraction wall.
Non-statutory notes and guidance:
Note: From Year 5, decimals and percentages are explicitly mentioned within the fractions section. Objectives that refer to either of these only have been removed and will be covered in another blog in this series.
For this lesson, I would like to address the following objective:
What excites me about this objective is that it is one of the few objectives that explicitly refers to the use of materials and diagrams to support understanding.
While it may be tempting to use the trick of turning a whole number multiplied by a fraction into a fraction of amount questions, it will not give the necessary understanding of what happens when a fraction is multiplied.
For this objective you would need to show fluency of performing operations such as 3 x ⅖. It should be one of the last objectives that you do teach in the sequence of objectives in Year 5 as it needs a deep understanding of fractions that I have not had time to go into detail such as mixed numbers, top-heavy fractions and converting between them.
When introducing multiplication with fractions, care needs to be given into how they are presented to the students. For example, 3 multiplied by ⅖ is difficult to visualise. But saying 3 lots of ⅖ helps us to see that we need to have 2/5 three times. Reminding students that multiplication is also repeated addition is a key part here. Rewriting the question as ⅖ + ⅖ + ⅖ = is helpful too.
In terms of using manipulatives, I believe it is best to use Cuisenaire rods to demonstrate how to do this. Below, I will detail how I would model this.

The orange rod represents our whole. We need to find which rod would represent a fifth.
Students should know that 5/5 make one whole so they need to find a rod that can be laid 5 times so that it is equal in length to the whole. It may be the case that students now instinctively know by this stage that the red rod represents 1/5. It is important that they lay 2 of the these to represent ⅖.
The next question we should ask will be is there a rod that is equivalent to 2/5. Students may need some time to do this but through experimentation or they will see that the purple rod can be one rod that represents ⅖.
At this point, ask the students to remove the 2 red blocks reminding them this block represents ⅖.
Refer back to the question of 3 x ⅖. Remind students that we need 3 lots of ⅖ and that if we have one lot of three already we will need 2 more lots. Tell students to place 2 more purple rods next to the first one. They should have the following:

Ask students how many 5ths we now have, reminding them that each purple block was worth ⅖. They will be able to count that there are ⁶⁄₅ altogether. Some students will be able to convert this to a mixed fraction. For those that cannot, ask them to place red blocks the length of the purple train, reminding them that red blocks here represent ⅕.
The students will have placed 6 red rods down. Ask the students if there are any rods that go further than the length of the whole and to move that number of rods and place them next to the whole. They should then have the following:
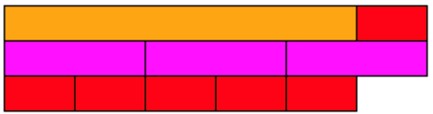
From here you can guide them in seeing that at the top train we have one whole and ⅕. So ⁶⁄₅ as a mixed fraction would be 1 ⅕.
Of course, it is then important to ensure that you get students to practice this with other questions using the I do, we do, you do approach. If using rods is new to you, then pre-planning these in advance and being absolutely clear in what you will do with the rods and the questions you will ask is crucial.
With novel content, it is best to keep the word problems simple and with numbers that students can understand. An appropriate word problem might be something like:
Amy is having a party with 3 other friends. Each person will eat ¾ of a pizza, how many pizzas will they need altogether?
First to note is that in total there are 4 people in total. Amy and 3 friends. The calculation will be 4 x ¾.
When using rods to demonstrate this, it is important to select a rod that can be split into four equal parts. The brown rod allows for this.
The process then follows much of the same as the previous example. Find the rods that denote a quarter and place three of them down.
The brown rod represents one whole pizza and the red rods (¾ of the total length) show how much each person intends to eat. Find a rod with the same value as the three red trains and place this down instead of the three red rods. This will be the dark green rod.
Remind students that 4 people will eat ¾ of a pizza, so we need 3 more dark green rods. Get the students to place them down.
Remind students that the brown rod represents one whole pizza and the total train of dark green rods, the total amount of pizza that will be consumed. Therefore, we need to place brown rods down until they are equal or just greater than the length of the dark green train. When students do this, they will get:

As each brown rod represents a whole pizza, it can be determined that 3 whole pizzas would be required.
As this is novel content, using it to see if students have misconceptions would be the best use of reasoning.
⅘ x 3 = ¹²⁄₁₅. Is this the correct answer? Explain why.
Here we are expecting the students to notice that the denominator has been multiplied by the whole number and so the answer would be incorrect as the denominator does not change.
The correct answer would be ¹²⁄₅ or 2 and ⅖
Non-statutory notes and guidance:
For this lesson, I want to focus on the following objective:
For me, this was easy to teach through simple algorithmic ways – multiply the numerators together; then multiply the denominators together; and finally write the fraction. But it gave very little conceptual understanding and failed to explain really why multiplying gave an answer that is smaller than the other two fractions. Much of a student’s experience has been that multiplication makes something bigger, when this is evidently not true.
We will deal with the question shown in the objective. ¼ x ½ =?
Teachers need to make careful pedagogical choices as to how they are going to pronounce this question. One third multiplied by one quarter invokes the area model of multiplication and this is what I will focus this on.
By this point, I am assuming that the students have had some prior knowledge working with rods.
First, we need to guide students through finding the unit whole. As the fractions we are multiplying are ¼ and ½, we want students to make an informed choice by choosing a rod that can be split equally into halves and quarters. As shown below, the brown rod allows for this to happen:
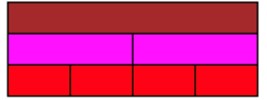
Next, we guide pupils into setting up the area model. We remind students that the unit whole is a brown rod and so the trains of the red rods and purple rods must equal the length of the brown rods.
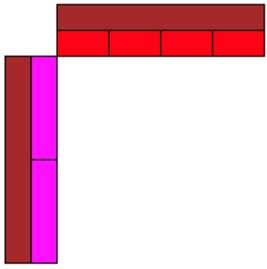
Here we check for understanding. Are students clear that the purple rods represent a half and the red rods represent a quarter? If not, then this needs to be reinforced.
As each side has a unit length of one whole, students now need to understand that the whole area created by the vertical and horizonal rods is equal to 1 x 1. In my experience, this is something that students struggle with the most and so it is worth spending some time looking at this. The shaded area below demonstrates this. That shaded area has a total value of 1.
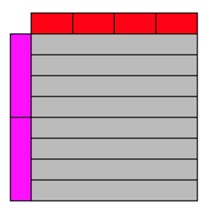
As we are looking at ¼ x ½, we only need to fill the area where one quarter and one half intersect. Which would be this.
Students can either use the red rods or the purple rods to complete the area of just one of the ½ rods and one of ¼ rods. Guide the students to this conclusion.
What is now important is to direct a pupils’ attention to the fact that from the area you have covered (the two purple rods you have just placed) you would need to replicate that eight more times to cover the whole area. I have tried to illustrate that below.
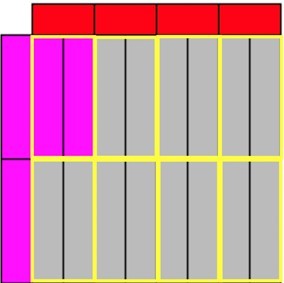
Remind pupils that the total area would be equal to one for the reasons set out above. Therefore, as the two purple rods would need to be replicated 8 times to fill the area but presently, it only covers up one part. The model shows that ¼ x ½ = ⅛.
A suitable problem might be the following:
There was ⅝ of a pie left in the fridge. Daniel ate ¼ of the leftover pie. How much of a pie did he have?
Students could represent that problem like this.
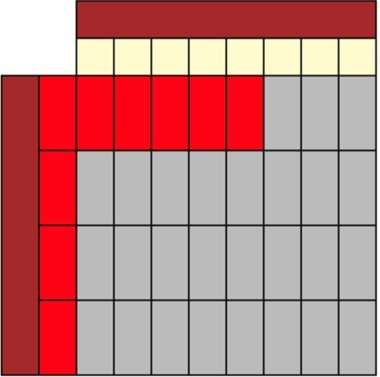
They would be able to see that there are 32 parts altogether and they layed 5 red rods down. Therefore, Dan has consumed ⁵⁄₃₂ of the leftover pie.
A suitable problem for Y6 might be the following:
⅔ x ¾ is the same as ¾ of ⅔. Prove it.
Students may create the following to prove that they are the same. The total area that is covered by each representation is 6 out of the 12 possible parts, making the answer ½.
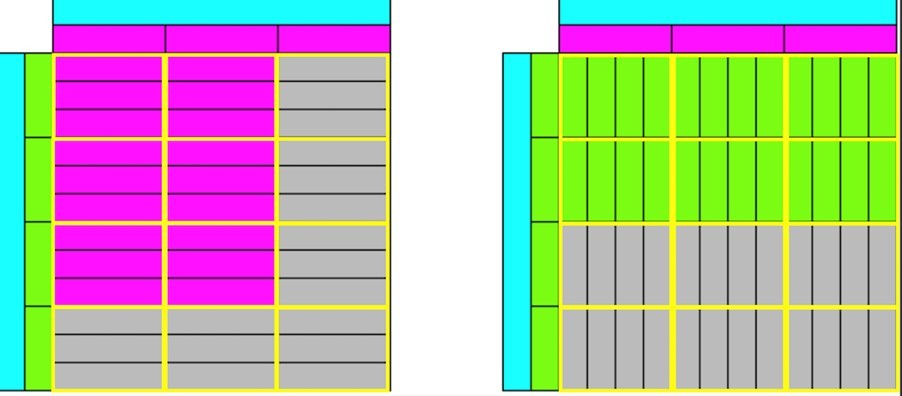
However, they can also rely on their knowledge of commutative law which always holds true for multiplication which is the following:
A x B = C and B x A = C
Whichever way you choose to multiply the numbers, you will always get the same answer.
Fractions is one of the most important topics in KS2 maths, and confidence in it is the key to succeeding at many other maths topics. Hopefully this post has shown you some ways to help your pupils achieve that confidence!
Every week Third Space Learning’s specialist primary maths tutors support thousands of students across hundreds of schools with weekly online 1 to 1 maths lessons designed to plug gaps and boost progress.
Since 2013 these personalised one to one lessons have helped over 169,000 primary and secondary students become more confident, able mathematicians.
Learn about the scaffolded lesson content or request a personalised quote for your school to speak to us about your school’s needs and how we can help.
![]()

How To Multiply Fractions: Step By Step Guide For Primary School Teachers & Pupils
5 min read
How To Add Fractions: Step By Step Guide For Primary School Teachers & Pupils
4 min read
What Is A Numerator And Denominator? Explained For Primary School Teachers & Pupils
3 min read
How To Divide Fractions: Step By Step Guide For Primary School Teachers & Pupils